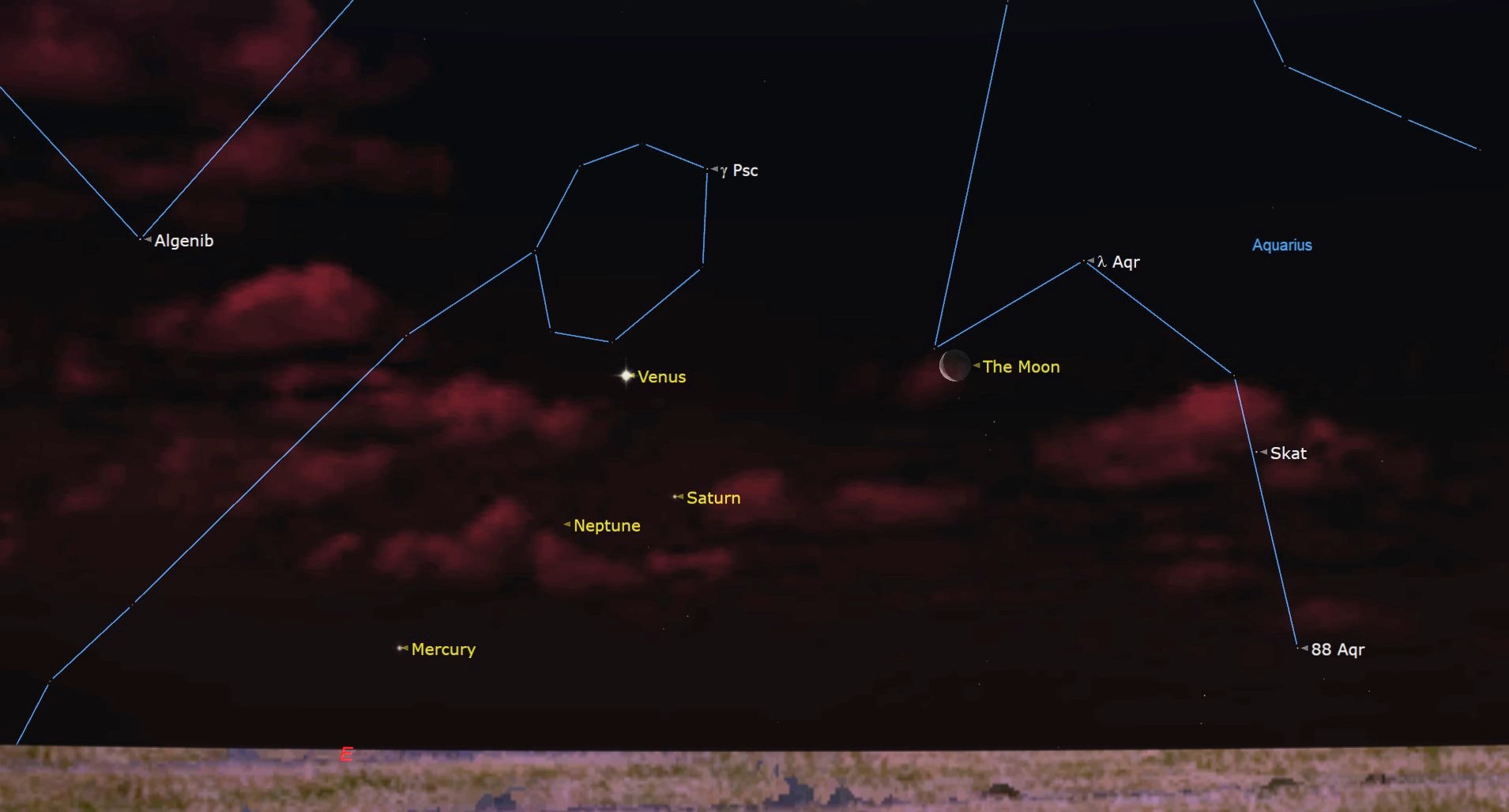
A universe without mathematics is beyond the scope of our imagination

This article was originally published at The Conversation. The publication contributed the article to Space.com's Expert Voices: Op-Ed & Insights.

Peter Watson, Emeritus professor, Physics, Carleton University

Almost 400 years ago, in The Assayer, Galileo wrote: "Philosophy is written in this grand book, the universe … [But the book] is written in the language of mathematics." He was much more than an astronomer, and this can almost be thought of as the first writing on the scientific method.

We do not know who first started applying mathematics to scientific study, but it is plausible that it was the Babylonians, who used it to discover the pattern underlying eclipses, nearly 3,000 years ago. But it took 2,500 years and the invention of calculus and Newtonian physics to explain the patterns.

Since then, probably every single major scientific discovery has used mathematics in some form, simply because it is far more powerful than any other human language. It is not surprising that this has led many people to claim that mathematics is much more: that the universe is created by a mathematician.

So could we imagine a universe in which mathematics does not work?

The language of mathematics

The Sapir-Whorf hypothesis asserts that you cannot discuss a concept unless you have the language to describe it.

In any science, and physics in particular, we need to describe concepts that do not map well on to any human language. One can describe an electron, but the moment we start asking questions like "What color is it?" we start to realize the inadequacies of English.

The color of an object depends on the wavelengths of light reflected by it, so an electron has no color, or more accurately, all colors. The question itself is meaningless. But ask "How does an electron behave?" and the answer is, in principle, simple. In 1928, Paul A.M. Dirac wrote down an equation that describes the behavior of an electron almost perfectly under all circumstances. This does not mean it is simple when we look at the details.

For example, an electron behaves as a tiny magnet. The magnitude can be calculated, but the calculation is horrendously complicated. Explaining an aurora, for example, requires us to understand orbital mechanics, magnetic fields and atomic physics, but at heart, these are just more mathematics.

But it is when we think of the individual that we realize that a human commitment to logical, mathematical thinking goes much deeper. The decision to overtake a slow-moving car does not involve the explicit integration of the equations of motion, but we certainly do it implicitly. A Tesla on autopilot will actually solve them explicitly.

Predicting chaos

So we really should not be surprised that mathematics is not just a language for describing the external world, but in many ways the only one. But just because something can be described mathematically does not mean it can be predicted.

One of the more remarkable discoveries of the last 50 years has been the discovery of "chaotic systems." These can be apparently simple mathematical systems that cannot be solved precisely. It turns out that many systems are chaotic in this sense. Hurricane tracks in the Caribbean are superficially similar to eclipse tracks, but we cannot predict them precisely with all the power of modern computers.

However, we understand why: the equations that describe weather are intrinsically chaotic, so we can make accurate predictions in the short term, (about 24 hours), but these become increasingly unreliable over days. Similarly, quantum mechanics provides a theory where we know precisely what predictions cannot be made precisely. One can calculate the properties of an electron very accurately, but we cannot predict what an individual one will do.

Hurricanes are obviously intermittent events, and we cannot predict when one will happen in advance. But the mere fact that we cannot predict an event precisely does not mean we cannot describe it when it happens. We can even handle one-off events: it is generally accepted that the universe was created in the Big Bang and we have a remarkably precise theory of that.

Designing social systems

A whole host of social phenomena, from the stock market to revolutions, lack good predictive mathematics, but we can describe what has happened and to some extent construct model systems.

So how about personal relationships? Love may be blind, but relationships are certainly predictable. The vast majority of us choose partners inside our social class and linguistic group, so there is absolutely no doubt that is true in the statistical sense. But it is also true in the local sense. A host of dating sites make their money by algorithms that at least make some pretense at matching you to your ideal mate.

A universe that could not be described mathematically would need to be fundamentally irrational and not merely unpredictable. Just because a theory is implausible does not mean we could not describe it mathematically.

But I do not think we live in that universe, and I suspect we cannot imagine a non-mathematical universe.

This article is republished from The Conversation under a Creative Commons license. Read the original article.

Follow all of the Expert Voices issues and debates — and become part of the discussion — on Facebook and Twitter. The views expressed are those of the author and do not necessarily reflect the views of the publisher.